They also realize that the more stable a particular nucleus is, the greater the amount of energy necessary to pull it apart. The binding energy is the energy that we must provide to disassemble a nucleus into its constituent nucleons. In this “thought-experiment” disassembly process, we assume that each individual neutron or proton is carefully removed from the parent nucleus and placed at rest out of range of any of the forces associated with the other nucleons. We can use equation 4.4 to calculate the binding energy (expressed in MeV) for any nucleus (except ordinary hydrogen, which contains only a single proton)
Binding Energy (MeV) = 931.5 [Z mH + (A – Z) mn – Matom] (4.4)
where Z is the atomic number (number of protons), mH is the mass of the hydrogen atom (in atomic mass units [amu]), A is the relative atomic mass (total number of nucleons) expressed in amu, mn is the mass of the neutron (expressed in amu), and Matom is the experimentally determined mass of the atom (in amu).
To demonstrate the importance of equation 4.4, we now use it to calculate the total binding energy of the helium-4 nucleus (see Figure 4.1). If we take 1.0078 amu as the mass of the hydrogen atom (mH), 1.0087 amu as the mass of the neutron, and 4.0026 amu as the mass of the helium atom, including its two orbiting electrons, the total binding energy of helium-4 is 28.3 MeV. Since the helium-4 nucleus contains two protons and two neutrons, the binding energy per nucleon is approximately 7.1 MeV. Scientists treat the binding energy per nucleon as a convenient figure of merit when they compare the characteristics of various nuclides.
As shown in Figure 4.8, the binding energy per nucleon reaches a maximum value of about 8.7 MeV per nucleon when A is about 60. This means that the nuclei of nickel-60 (60 28Ni), cobalt-59 (59 27Co), and iron-58 (58 26Fe) are much more tightly bound together than other nuclei with significantly higher or lower nucleon populations. For example, because of their relatively lower binding energy per nucleon, the heavy nuclei of uranium-235 and plutonium-239 readily support the process of nuclear fission. Other factors also influence the suitability of these two radioactive isotopes as nuclear fuels in both nuclear reactors and nuclear explosive devices.
Similarly, certain light nuclei (such as lithium-6, deuterium, and tritium) experience the process of nuclear fusion when placed in a proper, very high temperature (thermonuclear) environment. Both the fission and fusion nuclear reactions involve a general transformation of the reactant nucleus (or nuclei) from a lower binding energy per nucleon condition into a product nucleus (or nuclei) that exhibits a higher binding energy per nucleon. The change in binding energy (as expressed by equation 4.4) accounts for the liberation of an enormous amount of energy—typically, about 200 MeV per fission reaction.
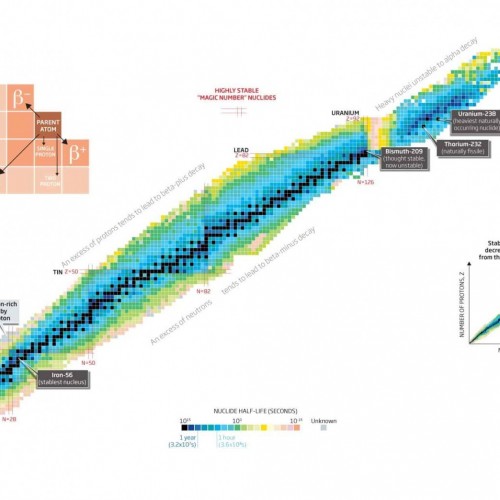Nuclear Stability
